To see the other types of publications on this topic, follow the link: Ising.
Dissertations / Theses on the topic 'Ising'
Create a spot-on reference in APA, MLA, Chicago, Harvard, and other styles
Consult the top 50 dissertations / theses for your research on the topic 'Ising.'
Next to every source in the list of references, there is an 'Add to bibliography' button. Press on it, and we will generate automatically the bibliographic reference to the chosen work in the citation style you need: APA, MLA, Harvard, Chicago, Vancouver, etc.
You can also download the full text of the academic publication as pdf and read online its abstract whenever available in the metadata.
Browse dissertations / theses on a wide variety of disciplines and organise your bibliography correctly.
1
Giokas, Philip. "Aspects of the Ising and tricritical Ising models." Thesis, King's College London (University of London), 2013. https://kclpure.kcl.ac.uk/portal/en/theses/aspects-of-the-ising-and-tricritical-ising-models(8bb2e06f-aef7-45ca-8058-3a3eea92b9e6).html.
Full textAbstract:
This thesis is concerned with several aspects of the Ising and tri-critical Ising models in two-dimensions. These are much-studied models relevant in both condensed matter physics as descriptions of the critical phenomena of two- dimensional systems and in String theory as building blocks of the string world sheet theory. The first part of the thesis is concerned with the derivation of differential equations for the critical four-point function in the Ising model. We present a method which provides the well-known standard solutions by a new and efficient route. The second part of the thesis is concerned with off-critical behaviour, and in particular the numerical study of perturbations of conformal field theory through the truncated conformal space approach. We show that the coupling constant undergoes significant renormalization in this scheme, and in particular the Ising model can be found as a fixed point for a finite value of the bare coupling constant. The renormalization group equations we find are of general use in the TCSA approach. The final part of the thesis considers off-critical boundary conditions in the tri-critical Ising model. We study them using a variant of the mean-field method and find a qualitative description of the space of boundary conditions that is in accord with the exact conformal field theory description. This is both a test of the method and its applicability in new domains, and also shows that previously published results are in error.
2
Li, Chengshu. "Tricritical Ising edge modes in a Majorana-Ising ladder." Thesis, University of British Columbia, 2017. http://hdl.handle.net/2429/62467.
Full textAbstract:
While Majorana fermions remain at large as fundamental particles, they emerge in condensed matter systems with peculiar properties. Grover et al. proposed a Majorana-Ising chain model, or the GSV model, where the system undergoes a tricritical Ising transition by tuning just one parameter. In this work, we generalize this model to a ladder with inter-chain Majorana couplings. From a mean field analysis, we argue that the tricritical Ising transition will also occur with inter-chain couplings that allow the system to be gapless in the non-interacting case. More crucially, based on analysis of the interacting chain model and the non-interacting ladder model, we expect the tricritical Ising modes to appear on the edges, a feature that might persist when going to 2d. We carry out extensive DMRG calculations to verify the theory in the ladder model. Finally, we discuss possible numerical probes of a 2d model.Science, Faculty of
Physics and Astronomy, Department of
Graduate
3
Karevski, Dragi. "Ising Quantum Chains." Habilitation à diriger des recherches, Université Henri Poincaré - Nancy I, 2005. http://tel.archives-ouvertes.fr/hal-00113500.
Full textAbstract:
The aim of this article is to give a pedagogical introduction to the exact equilibrium and nonequilibrium properties of free fermionic quantum spin chains. In a first part we present in full details the canonical diagonalisation procedure and review quickly the equilibrium dynamical properties. The phase diagram is analysed and possible phase transitions are discussed. The two next chapters are concerned with the effect of aperiodicity and quenched disorder on the critical properties of the quantum chain. The remaining part is devoted to the nonequilibrium dynamical behaviour of such quantum chains relaxing from a nonequilibrium pure initial state. In particular, a special attention is made on the relaxation of transverse magnetization. Two-time linear response functions and correlation functions are also considered, giving insights on the nature of the final nonequilibrium stationnary state. The possibility of aging is also discussed.
4
Kamenetsky, Dmitry, and dkamen@rsise anu edu au. "Ising Graphical Model." The Australian National University. ANU College of Engineering and Computer Science, 2010. http://thesis.anu.edu.au./public/adt-ANU20100727.221031.
Full textAbstract:
The Ising model is an important model in statistical physics, with over 10,000 papers published on the topic. This model assumes binary variables and only local pairwise interactions between neighbouring nodes. Inference for the general Ising model is NP-hard; this includes tasks such as calculating the partition function, finding a lowest-energy (ground) state and computing marginal probabilities. Past approaches have proceeded by working with classes of tractable Ising models, such as Ising models defined on a planar graph. For such models, the partition function and ground state can be computed exactly in polynomial time by establishing a correspondence with perfect matchings in a related graph.
In this thesis we continue this line of research. In particular we simplify previous inference algorithms for the planar Ising model. The key to our construction is the complementary correspondence between graph cuts of the model graph and perfect matchings of its expanded dual. We show that our exact algorithms are effective and efficient on a number of real-world machine learning problems. We also investigate heuristic methods for approximating ground states of non-planar Ising models. We show that in this setting our approximative algorithms are superior than current state-of-the-art methods.
5
Hystad, Grethe. "Periodic Ising Correlations." Diss., The University of Arizona, 2009. http://hdl.handle.net/10150/196130.
Full textAbstract:
We consider the finite two-dimensional Ising model on a lattice with periodic boundaryconditions. Kaufman determined the spectrum of the transfer matrix on the finite,periodic lattice, and her derivation was a simplification of Onsager's famous result onsolving the two-dimensional Ising model. We derive and rework Kaufman's resultsby applying representation theory, which give us a more direct approach to computethe spectrum of the transfer matrix. We determine formulas for the spin correlationfunction that depend on the matrix elements of the induced rotation associated withthe spin operator. The representation of the spin matrix elements is obtained byconsidering the spin operator as an intertwining map. We wrap the lattice aroundthe cylinder taking the semi-infinite volume limit. We control the scaling limit of themulti-spin Ising correlations on the cylinder as the temperature approaches the criticaltemperature from below in terms of a Bugrij-Lisovyy conjecture for the spin matrixelements on the finite, periodic lattice. Finally, we compute the matrix representationof the spin operator for temperatures below the critical temperature in the infinite-volume limit in the pure state defined by plus boundary conditions.
6
Varrassi, Lorenzo. "Il modello di Ising." Bachelor's thesis, Alma Mater Studiorum - Università di Bologna, 2016. http://amslaurea.unibo.it/10573/.
Full textAbstract:
L'elaborato fornisce una introduzione al modello di Ising, utilizzato nello studio delle transizioni di fase tra la fase ferromagnetica e quella paramagnetica dei materiali.
Nella prima parte viene trattato il modello unidimensionale, di cui viene esposta la soluzione esatta attraverso l'utilizzo delle matrici di trasferimento, dimostrando quindi l'inesistenza di una transizione di fase a temperature finite non nulle. Vengono calcolate le funzioni termodinamiche e se ne dimostra l'indipendenza dalle condizioni al contorno nel limite termodinamico.Viene proposta infine una spiegazione qualitativa del comportamento microscopico, attraverso la lunghezza di correlazione.
Nella seconda parte viene trattato il caso a due dimensioni. Inizialmente viene determinata la temperatura critica per reticoli quadrati, attraverso il riconoscimento della presenza di una relazione di dualita tra l'espansione per alte e per basse temperature della funzione di partizione. Successivamente si fornisce la soluzione esatta attraverso una versione modificata del procedimento, originariamente ideato da L.Onsager, di cui e proposta una traccia della dimostrazione.
Viene infine brevemente discussa l'importanza che questo risultato ebbe storicamente nella fisica delle transizioni di fase.
7
Malmini, Ranasinghe P. K. C. "Gonihedric 3D Ising models." Thesis, Heriot-Watt University, 1997. http://hdl.handle.net/10399/675.
Full text
8
Pugh, Mathew. "Ising model and beyond." Thesis, Cardiff University, 2008. http://orca.cf.ac.uk/54791/.
Full textAbstract:
We study the SU(3) AVE graphs, which appear in the classification of modular in variant partition functions from numerous viewpoints, including determination of their Boltzmann weights, representations of Hecke algebras, a new notion of A2 planar algebras and their modules, various Hilbert series of dimensions and spectral measures, and the K-theory of associated Cuntz-Krieger algebras. We compute the K-theory of the of the Cuntz-Krieger algebras associated to the SU(3) AVE graphs. We compute the numerical values of the Ocneanu cells, and consequently representations of the Hecke algebra, for the AVE graphs. Some such representations have appeared in the literature and we compare our results. We use these cells to define an SU(3) analogue of the Goodman-de la Harpe-Jones construction of a subfactor, where we embed the j42-Temperley-Lieb algebra in an AF path-algebra of the SU(3) AVE graphs. Using this construction, we realize all SU(3) modular invariants by subfactors previously announced by Ocneanu. We give a diagrammatic representation of the i42-Temperley-Lieb algebra, and show that it is isomorphic to Wenzl's representation of a Hecke algebra. Generalizing Jones's notion of a planar algebra, we construct an 42-planar algebra which captures the structure contained in the SU(3) AVE subfactors. We show that the subfactor for an AVE graph with a flat connection has a description as a flat >12-planar algebra. We introduce the notion of modules over an 42-planar algebra, and describe certain irreducible Hilbert A2- Temperley-Lieb-modules. A partial decomposition of the ,42-planar algebras for the AVE graphs is achieved. We compare various Hilbert series of dimensions associated to ADE models for SU(2), and the Hilbert series of certain Calabi-Yau algebras of dimension 3. We also consider spectral measures for the ADE graphs and generalize to SU(3), and in particular obtain spectral measures for the infinite SU(3) graphs.
9
Paula, Gilberto Luiz de Souza. "Modelos de Ising dinamico." reponame:Repositório Institucional da UFSC, 1994. http://repositorio.ufsc.br/xmlui/handle/123456789/76170.
Full textAbstract:
Dissertação (mestrado) - Universidade Federal de Santa Catarina, Centro de Ciencias Fisicas e MatematicasMade available in DSpace on 2012-10-16T07:56:41Z (GMT). No. of bitstreams: 0Bitstream added on 2016-01-08T18:59:08Z : No. of bitstreams: 1 96497.pdf: 1094541 bytes, checksum: cb7b6164b0d0951706f97e2c507d68c1 (MD5)
Nesta dissertação estudamos o comportamento dinâmico de dois modelos ferromagnéticos através da equação mestra. No primeiro deles consideramos o modelo de Ising num gradiente de temperatura e determinamos os estados estacionários através das prescrições de Metropolis e Glauber. Mostramos que na ausência de correlações os estados estacionários são diferentes, enquanto que levando-se em conta correlações entre primeiros vizinhos os estados estacionários são os mesmos. No segundo modelo, determinamos o diagrama de fases do modelo de Ising dinâmico em um campo aleatório na situação estacionária. Mostramos que os estados estacionários coincidem com os de equilíbrio para todo o espaço de parâmetros com exceção das vizinhanças das transições de primeira ordem.
10
Iberti, Massimo. "Ising-Kac models near criticality." Thesis, University of Warwick, 2018. http://wrap.warwick.ac.uk/109480/.
Full textAbstract:
The present thesis consists in an investigation around the result shown by H. Weber and J.C. Mourrat in [MW17a], where the authors proved that the fluctuation of an Ising models with Kac interaction under a Glauber-type dynamic on a periodic two-dimensional discrete torus near criticality converge to the solution of the Stochastic Quantization Equation Φ 4/2. In Chapter 2, starting from a conjecture in [SW16], we show the robustness of the method proving the convergence in law of the fluctuation field for a general class of ferromagnetic spin models with Kac interaction undergoing a Glauber dynamic near critical temperature. We show that the limiting law solves an SPDE that depends heavily on the state space of the spin system and, as a consequence of our method, we construct a spin system whose dynamical fluctuation field converges to Φ 2n/2. In Chapter 3 we apply an idea by H. Weber and P. Tsatsoulis employed in [TW16], to show tightness for the sequence of magnetization fluctuation fields of the Ising-Kac model on a periodic two-dimensional discrete torus near criticality and characterise the law of the limit as the Φ 4/2 measure on the torus. This result is not an immediate consequence of [MW17a]. In Chapter 4 we study the fluctuations of the magnetization field of the Ising-Kac model under the Kawasaki dynamic at criticality in a one dimensional discrete torus, and we provide some evidence towards the convergence in law to the solution to the Stochastic Cahn-Hilliard equation.
11
Dikkala, Sai Nishanth. "Testing properties of Ising models." Thesis, Massachusetts Institute of Technology, 2017. http://hdl.handle.net/1721.1/108844.
Full textAbstract:
Thesis: S.M., Massachusetts Institute of Technology, Department of Electrical Engineering and Computer Science, 2017.This electronic version was submitted by the student author. The certified thesis is available in the Institute Archives and Special Collections.
Cataloged from student-submitted PDF version of thesis.
Includes bibliographical references (pages 99-102).
Given samples from an unknown multivariate distribution p, is it possible to distinguish whether p is the product of its marginals versus p being [epsilon]-far from every product distribution? Similarly, is it possible to distinguish whether p equals a given distribution q versus p and q being [epsilon]-far from each other? These problems of testing independence and goodness-of- fit have received enormous attention in statistics, information theory, and theoretical computer science, with sample-optimal algorithms known in several interesting regimes of parameters [14, 15, 17, 18, 20]. Unfortunately, it has also been understood that these problems become intractable in large dimensions, necessitating exponential sample complexity. Motivated by the exponential lower bounds for general distributions as well as the ubiquity of Markov Random Fields (MRFs) in the modeling of high-dimensional distributions, we study distribution testing on structured multivariate distributions, and in particular the prototypical example of MRFs: the Ising Model. We demonstrate that, in this structured setting, we can avoid the curse of dimensionality, obtaining sample and time efficient testers for independence and goodness-of-fit which yield a sample complexity of poly(n)=[epsilon]2 on n-node Ising models. Along the way, we develop new tools for establishing concentration of functions of the Ising model, using the exchangeable pairs framework developed by Chatterjee [27], and improving upon this framework. In particular, we prove tighter concentration results for multi-linear functions of the Ising model in the high-temperature regime. We also prove a lower bound of n=[epsilon] on the sample complexity required for testing uniformity and independence of n-node Ising models.
by Sai Nishanth Dikkala.
S.M.
12
Silva, Romero Tavares da. "ALEATORIEDADE EM MODELOS DE ISING." Universidade de São Paulo, 1993. http://www.teses.usp.br/teses/disponiveis/43/43133/tde-22052012-133450/.
Full textAbstract:
Na primeira parte deste trabalho propomos uma aproximacão de campo médio dinâmico para analisar modelos de Ising com elementos e aleatoriedade definidos por distribuicões de probabilidades discretas. Analisamos o modelo com campo aleatório (S = 1/2), com interações aleatórias (S = 1/2), com diluição de sítios (S = 1/2) e com anisotropia aleatória (S = 1), obtendo os respectivos diagramas de fases. Na segunda parte analisamos modelos de vidros de spin (S= 3/2) com anisotropia de campo cristalino. Estudamos o modelo de van Hemmen, e o modelo clássico à la Sherrington e Kirkpatrick dentro do esquema de réplicas simétricas, obtendo os diagramas de fases correspondentes.In the first part of this work we propose a dynamical mean field approximation to analyse Ising models with elements of randomnss, defined by discret probability functions. We have analysed the random field model (S = 1/2); the random bond model (S = 1/2); the site diluted model (S = 3/2) and the random crystal field model (S = 1), obtaining the respective phase diagrams. In the second part we have analysed spinglass models (S = 3/2) in the presence of a crystal field. We have studied the van Hemmen and the classic spin glass model à la Sherrington and Kirkpatrick, using replica symmetric scheme, to obtain the corresponding phase diagrams.
13
Tamashiro, Mário Noboru. "Modelos de Ising com Competição." Universidade de São Paulo, 1996. http://www.teses.usp.br/teses/disponiveis/43/43133/tde-28022014-163442/.
Full textAbstract:
Neste trabalho consideramos três modelos de Ising com competição: que é gerada por acoplamentos dinâmicos de caráter antagônicos, pela própria geometria da rede subjacente ou através de interações de periodicidades uniaxiais competitivas e elementos de desordem. O primeiro modelo, no qual as técnicas de mecânica estatística de equilíbrio não se aplicam, consiste numa rede neural atratora completamente conectada com acoplamentos assimétricos armazenando p = 2 padrões, cuja evolução temporal pode ser descrita (no caso de atualização síncrona) por um mapeamento dissipativo bidimensional. O segundo modelo se refere ao problema clássico do antiferromagneto de Ising na rede triangular na presença de um campo magnético uniforme, investigado através de diversas aproximações - em particular, através de uma aproximação de Bethe-Peierls considerando três sub-redes interpenetrantes equivalentes. O terceiro modelo, introduzido para investigar o efeito de uma desordem congelada em um sistema magnético modulado, é definido pelo modelo ANNNI em um campo aleatório. Inicialmente consideramos um análogo deste modelo na árvore de Cayley, no limite de coordenação infinita, que pode ser formulado em termos de um mapeamento dissipativo bidimensional. A seguir, consideramos uma versão de campo médio em uma rede cúbica simples. que permite uma análise das superfícies de transição de primeira ordem e das linhas tricriticas.In this work we consider three Ising models with competition: which is generated by dynamical couplings of antagonistic character, by the geometry of the underlying lattice, or by interactions of competitive uniaxial periodicities and disorder elements. The first model, for which equilibrium statistical mechanics techniques do not apply, consists in a fully connected attractor neural network storing p = 2 patterns, whose temporal evolution can be described (in the case of synchronous updating) by a two-dimensional dissipative mapping. The second model refers to the classic problem of the Ising antiferromagnet on the triangular lattice in the presence of a uniform magnetic field, which is investigated by various approximations - in particular, by a Bethe-Peierls approximation considering three interpenetrating equivalent sublattices. The third model, introduced to investigate the effects of quenched disorder in a modulated magnetic system, is defined by the ANNNI model in a random field. Initially we consider an analogous of this model on a Cayley tree, in the infinite-coordination limit, which can be formulated in terms of a two-dimensional dissipative mapping. Next, we consider a mean-field version on a simple cubic lattice, which allows for an analysis of the first-order transition surfaces and tricritical lines.
14
Marsolais, Annette M. "The Equivalence Between the Kitaev, the Transverse Quantum Ising Model and the Classical Ising Model." University of Akron / OhioLINK, 2021. http://rave.ohiolink.edu/etdc/view?acc_num=akron1619792923386843.
Full text
15
Ridderstolpe, Ludwig. "Exact Solutions of the Ising Model." Thesis, Uppsala universitet, Teoretisk astrofysik, 2017. http://urn.kb.se/resolve?urn=urn:nbn:se:uu:diva-329081.
Full textAbstract:
This report presents the general Ising model and its basic assumptions. This study aims to, from diagonalization of the Transfer Matrix, obtain the Helmholtz free energy and the exclusion of a phase transition for the one-dimensional Ising model under an external magnetic field. Furthermore from establishing the commutation relations of the Transfer matrices and using the Kramers-Wannier duality one finds the free energy and the presence of a phase transition for the square-lattice Ising model.
16
Lacasse, Martin Daniel. "Exact dynamics of small Ising systems." Thesis, McGill University, 1994. http://digitool.Library.McGill.CA:80/R/?func=dbin-jump-full&object_id=28814.
Full textAbstract:
Monte Carlo simulations used for representing dynamical physical phenomena are studied in terms of a Markov chain operator acting on the probability distrubution of the states of a given system. The most general transition rule satisfying detailed balance and leading to a canonical ensemble probability distribution is derived using this formalism. The explicit Markov chain representing the two most commonly used canonical algorithms, the Metropolis and the Glauber transition rules, is then constructed and numerically applied to the states of an Ising model. The dynamical properties of the system are studied for each algorithm. Various measures, such as time-time correlation functions, are estimated for different system sizes and finite-size sealing is applied. In particular, the effects of the transition rule on the dynamic critical exponent is investigated.We at first examine one- and two-dimensional systems using periodic boundary conditions. Systems with free boundary conditions were also studied, and their results were equivalent with respect to the dynamical critical properties of the system. The effects of conservation laws were also investigated and both conserved and non-conserved systems were studied. Both local and non-local spin-exchange dynamics were investigated for conserved systems. Finally, our approach was used to simulate quenches on small systems.
This method is them used to analyze phenomenological transformations done by dynamical renormalization-group (RG) methods. It is found that, when the RG transformation is linear in probability space, there exists a corresponding Markov chain generating the time sequence of the renormalized systems. An example is given for the one-dimensional Ising model.
17
Smith, Thomas H. R. "Driven interfaces in the Ising model." Thesis, University of Bristol, 2010. http://ethos.bl.uk/OrderDetails.do?uin=uk.bl.ethos.535182.
Full text
18
Gray, Sean. "Bootstrapping the Three-dimensional Ising Model." Thesis, Uppsala universitet, Teoretisk fysik, 2017. http://urn.kb.se/resolve?urn=urn:nbn:se:uu:diva-322146.
Full textAbstract:
This thesis begins with the fundamentals of conformal field theory in three dimensions. The general properties of the conformal bootstrap are then reviewed. The three-dimensional Ising model is presented from the perspective of the renormalization group, after which the conformal field theory aspect at the critical point is discussed. Finally, the bootstrap programme is applied to the three-dimensional Ising model using numerical techniques, and the results analysed.
19
Milanesi, Paolo. "Sur la métastabilité de la dynamique de Glauber." Thesis, Aix-Marseille, 2018. http://www.theses.fr/2018AIXM0663.
Full textAbstract:
Dans cette thèse on étudie le comportement métastable de la dynamique de Glauber pour le modèle d'Ising en dimension deux, dans le régime où la température est fixée à une valeur sous critique et le champ magnétique extérieur est très petit. En volume infini, ce modèle a été étudié par Schonmann et Shlosman qui montrent le lien existant entre le temps moyen de transition et la tension de surface intégrée de la forme de Wulff. Cependant, l'exponentialité du temps de transition, déjà en volume fini, reste un problème ouvert. Dans cette thèse on adresse cette question. On donne d'abord un cadre théorique pour traiter ces dynamiques markoviennes métastable pour lesquelles le support de la mesure métastable n'est pas réductible à une seule configuration. Nos techniques permettent d'obtenir la loi exponentielle du temps de transition ainsi que d'estimer sa moyenne et le temps de relaxation de la dynamique. Dans la deuxième partie de notre travail on s'adresse à la dynamique de Glauber métastable; on donne les bonnes définitions des ensemble métastable et stable et on estime les temps de relaxation des dynamiques restreintes à ces deux ensembles. Cela nous permet de mettre en œuvre les techniques étudiées dans la première partie du travail. Nos résultats sont vrais pour toute température sous critique et pour une grande classe de mesure de départIn this thesis we study the metastable behavior of the Glauber dynamics for the two-dimensional Ising model in the regime where the temperature is kept fixed at some subcritical value and the external magnetic field is vanishing.In the infinite volume regime, this model has been studied by Schonmann and Shlosman who show the connection between the mean transition time and the integrated surface tension of the Wulff shape. However, the exponentiality of the transition time, already in the finite volume case, is still an open problem. In this thesis we address this question.First, we give a theoretical framework to deal with metastable markovian dynamics such that the support of the metastable measure is not reducible to a single configuration. Our techniques allow to get the exponential law of the transition time as well as to estimate its mean and the relaxation time of the dynamics. In the second part of the thesis we address the metastable Glauber dynamics; we give suitable definitions of the metastable and the stable sets and we estimate the relaxation time of the dynamics restricted to these two sets. In doing so, we are in good shape to exploit the techniques studied in the first part of our work. Our results hold true for any subcritical temperature and a wide class of starting measures
20
Pomponio, Octavio. "Transizioni di fase nel modello di Ising." Bachelor's thesis, Alma Mater Studiorum - Università di Bologna, 2015. http://amslaurea.unibo.it/9562/.
Full textAbstract:
L'elaborato tratta le transizioni di fase nel modello di Ising, usato per descrivere i sistemi magnetici. Tramite l'argomento di Landau viene introdotto il problema della dimensionalità per l'esistenza di una fase ferromagnetica. Con il sistema di un gas forzato su reticolo viene presentato il carattere universale dei fenomeni critici per mezzo degli esponenti critici. Viene poi risolto in modo esatto il modello unidimensionale, che non prevede una fase ferromagnetica. Per sistemi a dimensionali maggiore viene introdotto il metodo dell'approssimazione di campo medio. Viene infine determinato il valore della temperatura critica per reticoli planari quadrati e di questi viene mostrata la soluzione esatta di Lars Onsager.
21
Hausmann, Johannes. "Statistical mechanics of strongly driven Ising systems." [S.l.] : [s.n.], 2001. http://deposit.ddb.de/cgi-bin/dokserv?idn=963559311.
Full text
22
Zhang, Xingjun. "Critical Properties of Small World Ising Models." MSSTATE, 2005. http://sun.library.msstate.edu/ETD-db/theses/available/etd-11102005-220554/.
Full textAbstract:
In this dissertation, the critical scaling behavior of magnetic Ising models with long range interactions is studied. These long range interactions, when imposed in addition to interactions on a regular lattice, lead to small-world graphs. By using large-scale Monte Carlo simulations, together with finite-size scaling, the critical behavior of a number of different models is obtained. The Ising models studied in this dissertation include the z-model introduced by Scalettar, standard small-world bonds superimposed on a square lattice, and physical small-world bonds superimposed on a square lattice. From the scaling results of the Binder 4th order cumulant, the order parameter, and the susceptibility, the long-range interaction is found to drive the systems behavior from Ising-like to mean field, and drive the critical point to a higher temperature. It is concluded that with a large amount of strong long-range connections (compared to the interactions on regular lattices), so the long-range connection density is non-vanishing, systems have mean field behavior. With a weak interaction that vanishes for an infinite system size or for vanishing density of long-range connections the systems have Ising-like critical behavior. The crossover from Ising-like to mean-field behavior due to weak long-range interactions for systems with a large amount of long-range connections is also discussed. These results provide further evidence to support the existence of physical (quasi-) small-world nanomaterials.
23
Sakellariou, Jason. "Inverse inference in the asymmetric Ising model." Phd thesis, Université Paris Sud - Paris XI, 2013. http://tel.archives-ouvertes.fr/tel-00869738.
Full textAbstract:
Recent experimental techniques in biology made possible the acquisition of overwhelming amounts of data concerning complex biological networks, such as neural networks, gene regulation networks and protein-protein interaction networks. These techniques are able to record states of individual components of such networks (neurons, genes, proteins) for a large number of configurations. However, the most biologically relevantinformation lies in their connectivity and in the way their components interact, information that these techniques aren't able to record directly. The aim of this thesis is to study statistical methods for inferring information about the connectivity of complex networks starting from experimental data. The subject is approached from a statistical physics point of view drawing from the arsenal of methods developed in the study of spin glasses. Spin-glasses are prototypes of networks of discrete variables interacting in a complex way and are widely used to model biological networks. After an introduction of the models used and a discussion on the biological motivation of the thesis, all known methods of network inference are introduced and analysed from the point of view of their performance. Then, in the third part of the thesis, a new method is proposed which relies in the remark that the interactions in biology are not necessarily symmetric (i.e. the interaction from node A to node B is not the same as the one from B to A). It is shown that this assumption leads to methods that are both exact and efficient. This means that the interactions can be computed exactly, given a sufficient amount of data, and in a reasonable amount of time. This is an important original contribution since no other method is known to be both exact and efficient.
24
Cornell, Stephen John. "Studies of freezing in kinetic Ising models." Thesis, University of Oxford, 1990. http://ethos.bl.uk/OrderDetails.do?uin=uk.bl.ethos.257825.
Full text
25
Brown, A. S. "Critical phenomena in the Random Ising Model." Thesis, University of Edinburgh, 1986. http://ethos.bl.uk/OrderDetails.do?uin=uk.bl.ethos.370906.
Full text
26
Dinóla, Isabel Cristina Souza. "Super Antiferromagneto de Ising com campo uniforme." Universidade Federal do Amazonas, 2009. http://tede.ufam.edu.br/handle/tede/4543.
Full textAbstract:
Submitted by Geyciane Santos (geyciane_thamires@hotmail.com) on 2015-08-06T14:07:38Z
No. of bitstreams: 1
Dissertação - Isabel Cristina Souza Dinóla.pdf: 562066 bytes, checksum: fb6aeda0c4854690f169abad1c217b8d (MD5)Approved for entry into archive by Divisão de Documentação/BC Biblioteca Central (ddbc@ufam.edu.br) on 2015-08-07T13:20:19Z (GMT) No. of bitstreams: 1 Dissertação - Isabel Cristina Souza Dinóla.pdf: 562066 bytes, checksum: fb6aeda0c4854690f169abad1c217b8d (MD5)
Approved for entry into archive by Divisão de Documentação/BC Biblioteca Central (ddbc@ufam.edu.br) on 2015-08-07T13:24:35Z (GMT) No. of bitstreams: 1 Dissertação - Isabel Cristina Souza Dinóla.pdf: 562066 bytes, checksum: fb6aeda0c4854690f169abad1c217b8d (MD5)
Made available in DSpace on 2015-08-07T13:24:35Z (GMT). No. of bitstreams: 1 Dissertação - Isabel Cristina Souza Dinóla.pdf: 562066 bytes, checksum: fb6aeda0c4854690f169abad1c217b8d (MD5) Previous issue date: 2009-05-22
CAPES - Coordenação de Aperfeiçoamento de Pessoal de Nível Superior
The phase diagram of the two-dimensional super-antiferromagnetic (SAF) Ising model in the presence of a magnetic field is investigated within the framework of a real-space renormalization-group approximation. We consider nearest neighbor ferromagnetic interactions along the x(y) direction and antiferromagnetic interactions in the y(x) direction. The system presents a ordered phase at low temperatures and zero fields. The presence of a magnetic field induces a competition between the energy interactions of the SAF Hamiltonian. The resulting behavior has been a matter of controversy in the last years. We depicted the main results in the magnetic field versus temperature phase diagram. A second-order transition line separates a super-antiferromagnetic phase from a field induced ferromagnetic phase. Our study reveals that the magnetic field induces a phase transition at a single temperature value, thus, we did not find any evidence of reentrant behavior as claimed by some authors.
Utilizamos uma técnica de grupo de renormalização no espaço real para estudar o sistema super antiferromagneto (SAF) de Ising bidimensional sob a influência de um campo magnético externo. Neste modelo as interações de primeiros vizinhos na direção x são ferromagnéticas e na direção y são antiferromagnéticas. Este sistema apresenta uma fase ordenada, para baixas temperaturas e campos nulos, com uma estrutura de linhas ferromagnéticas e colunas antiferromagnéticas. A aplicação do campo magnético induz uma competição entre as energias de interação do modelo e o comportamento resultante desta competição tem sido objeto de estudo e gerado algumas controvérsias nos últimos anos. Na presença do campo magnético observa-se, além da fase SAF, a fase ferromagnética induzida pelo campo (FIC). Apresentamos neste trabalho o diagrama de fases completo do sistema SAF no plano temperatura versus campo magnético. O diagrama de fases obtido mostra uma linha de transição de segunda ordem separando a fase SAF da fase FIC. Nossos resultados contrariam resultados anteriores que preveêm um comportamento reentrante no diagrama de fases do sistema SAF.
27
Feng, Shuangtong. "Efficient Parallelization of 2D Ising Spin Systems." Thesis, Virginia Tech, 2001. http://hdl.handle.net/10919/36263.
Full textAbstract:
The problem of efficient parallelization of 2D Ising spin systems requires realistic algorithmic design and implementation based on an understanding of issues from computer science and statistical physics. In this work, we not only consider fundamental parallel computing issues but also ensure that the major constraints and criteria of 2D Ising spin systems are incorporated into our study. This realism in both parallel computation and statistical physics has rarely been reflected in previous research for this problem.
In this thesis,we designed and implemented a variety of parallel algorithms for both sweep spin selection and random spin selection. We analyzed our parallel algorithms on a portable and general parallel machine model, namely the LogP model. We were able to obtain rigorous theoretical run-times on LogP for all the parallel algorithms. Moreover, a guiding equation was derived for choosing data layouts (blocked vs. stripped) for sweep spin selection. In regards to random spin selection, we were able to develop parallel algorithms with efficient communication schemes. We analyzed randomness of our schemes using statistical methods and provided comparisons between the different schemes. Furthermore, algorithms were implemented and performance data gathered and analyzed in order to determine further design issues and validate theoretical analysis.
Master of Science
28
Björnberg, Jakob Erik. "Graphical representations of Ising and Potts models stochastic geometry of the quantum Ising model and the space-time Potts model /." Stockholm : Skolan för teknikvetenskap, Kungliga Tekniska högskolan, 2009. http://urn.kb.se/resolve?urn=urn:nbn:se:kth:diva-11267.
Full text
29
Björnberg, Jakob Erik. "Graphical representations of Ising and Potts models : Stochastic geometry of the quantum Ising model and the space-time Potts model." Doctoral thesis, KTH, Matematik (Inst.), 2009. http://urn.kb.se/resolve?urn=urn:nbn:se:kth:diva-11267.
Full text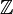Abstract:
HTML clipboard Statistical physics seeks to explain macroscopic properties of matter in terms of microscopic interactions. Of particular interest is the phenomenon of phase transition: the sudden changes in macroscopic properties as external conditions are varied. Two models in particular are of great interest to mathematicians, namely the Ising model of a magnet and the percolation model of a porous solid. These models in turn are part of the unifying framework of the random-cluster representation, a model for random graphs which was first studied by Fortuin and Kasteleyn in the 1970’s. The random-cluster representation has proved extremely useful in proving important facts about the Ising model and similar models. In this work we study the corresponding graphical framework for two related models. The first model is the transverse field quantum Ising model, an extension of the original Ising model which was introduced by Lieb, Schultz and Mattis in the 1960’s. The second model is the space–time percolation process, which is closely related to the contact model for the spread of disease. In Chapter 2 we define the appropriate space–time random-cluster model and explore a range of useful probabilistic techniques for studying it. The space– time Potts model emerges as a natural generalization of the quantum Ising model. The basic properties of the phase transitions in these models are treated in this chapter, such as the fact that there is at most one unbounded fk-cluster, and the resulting lower bound on the critical value in 
. In Chapter 3 we develop an alternative graphical representation of the quantum Ising model, called the random-parity representation. This representation is based on the random-current representation of the classical Ising model, and allows us to study in much greater detail the phase transition and critical behaviour. A major aim of this chapter is to prove sharpness of the phase transition in the quantum Ising model—a central issue in the theory— and to establish bounds on some critical exponents. We address these issues by using the random-parity representation to establish certain differential inequalities, integration of which gives the results. In Chapter 4 we explore some consequences and possible extensions of the results established in Chapters 2 and 3. For example, we determine the critical point for the quantum Ising model in 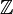and in ‘star-like’ geometries.HTML clipboard Statistisk fysik syftar till att förklara ett materials makroskopiska egenskaper i termer av dess mikroskopiska struktur. En särskilt intressant egenskap är är fenomenet fasövergång, det vill säga en plötslig förändring i de makroskopiska egenskaperna när externa förutsättningar varieras. Två modeller är särskilt intressanta för en matematiker, nämligen Ising-modellen av en magnet och perkolationsmodellen av ett poröst material. Dessa två modeller sammanförs av den så-kallade fk-modellen, en slumpgrafsmodell som först studerades av Fortuin och Kasteleyn på 1970-talet. fk-modellen har sedermera visat sig vara extremt användbar för att bevisa viktiga resultat om Ising-modellen och liknande modeller. I den här avhandlingen studeras den motsvarande grafiska strukturen hos två näraliggande modeller. Den första av dessa är den kvantteoretiska Isingmodellen med transverst fält, vilken är en utveckling av den klassiska Isingmodellen och först studerades av Lieb, Schultz och Mattis på 1960-talet. Den andra modellen är rumtid-perkolation, som är nära besläktad med kontaktmodellen av infektionsspridning. I Kapitel 2 definieras rumtid-fk-modellen, och flera probabilistiska verktyg utforskas för att studera dess grundläggande egenskaper. Vi möter rumtid-Potts-modellen, som uppenbarar sig som en naturlig generalisering av den kvantteoretiska Ising-modellen. De viktigaste egenskaperna hos fasövergången i dessa modeller behandlas i detta kapitel, exempelvis det faktum att det i fk-modellen finns högst en obegränsad komponent, samt den undre gräns för det kritiska värdet som detta innebär. I Kapitel 3 utvecklas en alternativ grafisk framställning av den kvantteoretiska Ising-modellen, den så-kallade slumpparitetsframställningen. Denna är baserad på slumpflödesframställningen av den klassiska Ising-modellen, och är ett verktyg som låter oss studera fasövergången och gränsbeteendet mycket närmare. Huvudsyftet med detta kapitel är att bevisa att fasövergången är skarp—en central egenskap—samt att fastslå olikheter för vissa kritiska exponenter. Metoden består i att använda slumpparitetsframställningen för att härleda vissa differentialolikheter, vilka sedan kan integreras för att lägga fast att gränsen är skarp. I Kapitel 4 utforskas några konsekvenser, samt möjliga vidareutvecklingar, av resultaten i de tidigare kapitlen. Exempelvis bestäms det kritiska värdet hos den kvantteoretiska Ising-modellen på
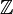, samt i ‘stjärnliknankde’ geometrier.QC 20100705
30
Björnberg, Jakob Erik. "Graphical representations of Ising and Potts models : stochastic geometry of the quantum Ising model and the space-time Potts model." Thesis, University of Cambridge, 2010. https://www.repository.cam.ac.uk/handle/1810/224774.
Full textAbstract:
Statistical physics seeks to explain macroscopic properties of matter in terms of microscopic interactions. Of particular interest is the phenomenon of phase transition: the sudden changes in macroscopic properties as external conditions are varied. Two models in particular are of great interest to mathematicians, namely the Ising model of a magnet and the percolation model of a porous solid. These models in turn are part of the unifying framework of the random-cluster representation, a model for random graphs which was first studied by Fortuin and Kasteleyn in the 1970's. The random-cluster representation has proved extremely useful in proving important facts about the Ising model and similar models. In this work we study the corresponding graphical framework for two related models. The first model is the transverse field quantum Ising model, an extension of the original Ising model which was introduced by Lieb, Schultz and Mattis in the 1960's. The second model is the space-time percolation process, which is closely related to the contact model for the spread of disease. In Chapter 2 we define the appropriate 'space-time' random-cluster model and explore a range of useful probabilistic techniques for studying it. The space-time Potts model emerges as a natural generalization of the quantum Ising model. The basic properties of the phase transitions in these models are treated in this chapter, such as the fact that there is at most one unbounded fk-cluster, and the resulting lower bound on the critical value in Z. In Chapter 3 we develop an alternative graphical representation of the quantum Ising model, called the random-parity representation. This representation is based on the random-current representation of the classical Ising model, and allows us to study in much greater detail the phase transition and critical behaviour. A major aim of this chapter is to prove sharpness of the phase transition in the quantum Ising model - a central issue in the theory - and to establish bounds on some critical exponents. We address these issues by using the random-parity representation to establish certain differential inequalities, integration of which give the results. In Chapter 4 we explore some consequences and possible extensions of the results established in Chapters 2 and 3. For example, we determine the critical point for the quantum Ising model in Z and in 'star-like' geometries.
31
Andrén, Daniel. "On the Ising problem and some matrix operations." Doctoral thesis, Umeå universitet, Institutionen för matematik och matematisk statistik, 2007. http://urn.kb.se/resolve?urn=urn:nbn:se:umu:diva-1129.
Full textAbstract:
The first part of the dissertation concerns the Ising problem proposed to Ernst Ising by his supervisor Wilhelm Lenz in the early 20s. The Ising model, or perhaps more correctly the Lenz-Ising model, tries to capture the behaviour of phase transitions, i.e. how local rules of engagement can produce large scale behaviour. Two decades later Lars Onsager solved the Ising problem for the quadratic lattice without an outer field. Using his ideas solutions for other lattices in two dimensions have been constructed. We describe a method for calculating the Ising partition function for immense square grids, up to linear order 320 (i.e. 102400 vertices). In three dimensions however only a few results are known. One of the most important unanswered questions is at which temperature the Ising model has its phase transition. In this dissertation it is shown that an upper bound for the critical coupling Kc, the inverse absolute temperature, is 0.29 for the tree dimensional cubic lattice. To be able to get more information one has to use different statistical methods. We describe one sampling method that can use simple state generation like the Metropolis algorithm for large lattices. We also discuss how to reconstruct the entropy from the model, in order to obtain parameters as the free energy. The Ising model gives a partition function associated with all finite graphs. In this dissertation we show that a number of interesting graph invariants can be calculated from the coefficients of the Ising partition function. We also give some interesting observations about the partition function in general and show that there are, for any N, N non-isomorphic graphs with the same Ising partition function. The second part of the dissertation is about matrix operations. We consider the problem of multiplying them when the entries are elements in a finite semiring or in an additively finitely generated semiring. We describe a method that uses O(n3 / log n) arithmetic operations. We also consider the problem of reducing n x n matrices over a finite field of size q using O(n2 / logq n) row operations in the worst case.
32
McKenzie, Ryan. "Fluctuations and phase transitions in quantum Ising systems." Thesis, University of British Columbia, 2016. http://hdl.handle.net/2429/59105.
Full textAbstract:
The quantum Ising model is perhaps the simplest possible model of a quantum magnetic material. Despite its simplicity, its versatility and wide range of applications, from quantum computation, to combinatorial optimization, to biophysics, make it one of the most important models of modern physics. In this thesis, we develop a general framework for studying quantum Ising systems with an arbitrary single ion Hamiltonian, with emphasis on the effects of quantum fluctuations, and the quantum phase transition between paramagnetic and ferromagnetic states that occurs when a magnetic field is applied transverse to the easy axis of the system. The magnetic insulating crystal LiHoF₄ is a physical realization of the quantum Ising model, with the additional features that the dominant coupling between spins is the long range dipolar interaction, and each electronic spin is strongly coupled to a nuclear degree of freedom. These nuclear degrees of freedom constitute a spin bath environment acting on the system. In this thesis, we present an effective low temperature Hamiltonian for LiHoF₄ that incorporates both these features, and we analyze the effects of the nuclear spin bath on the system. We find the lowest energy crystal field excitation in the system is gapped at the quantum critical point by the presence of the nuclear spins, with spectral weight being transferred down to a lower energy electronuclear mode that fully softens to zero at the quantum critical point. Furthermore, we present a toy model, the spin half spin half model, that illustrates the effects of an anisotropic hyperfine interaction on a quantum Ising system. We find the critical transverse field is increased when the longitudinal hyperfine coupling is dominant, as well as an enhancement of both the longitudinal electronic susceptibility and an applied longitudinal field. In addition, we present a field theoretic formalism for incorporating the effects of fluctuations beyond the random phase approximation in general quantum Ising systems. We find that any regular on site interaction, such as a nuclear spin bath, does not fundamentally alter the critical properties of a quantum Ising system. This formalism is used to calculate corrections to the magnetization of LiHoF₄.Science, Faculty of
Physics and Astronomy, Department of
Graduate
33
Andrén, Daniel. "On the Ising problem and some matrix operations /." Umeå : Dept. of Mathematics and Mathematical Statistics, Umeå University, 2007. http://urn.kb.se/resolve?urn=urn:nbn:se:umu:diva-1129.
Full text
34
Latremoliere, Franck Thierry. "Boundaries and interfaces in the planar ising ferromagnet." Thesis, University of Oxford, 1996. http://ethos.bl.uk/OrderDetails.do?uin=uk.bl.ethos.320674.
Full text
35
Canning, Andrew Magnus. "Ising spin models of partially connected neural networks." Thesis, University of Edinburgh, 1988. http://hdl.handle.net/1842/13304.
Full text
36
Hernandez, Hernandez Fabio 1990. "Estados de impureza no modelo de Ising quântico." [s.n.], 2016. http://repositorio.unicamp.br/jspui/handle/REPOSIP/322412.
Full textAbstract:
Orientador: Guillermo Gerardo Cabrera OyarzúnDissertação (mestrado) - Universidade Estadual de Campinas, Instituto de Física Gleb Wataghin
Made available in DSpace on 2018-08-30T18:12:28Z (GMT). No. of bitstreams: 1 Hernandez_FabioHernandez_M.pdf: 2178317 bytes, checksum: 00e18623b835112b2aa5c348e4651b65 (MD5) Previous issue date: 2016
Resumo: A descrição da dinâmica quântica de sistemas de muitos corpos é um ingrediente chave para computação e simulações quânticas. No presente projeto, estudamos a dinâmica de cadeias de spin na presença de impurezas ou defeitos. O sistema de Ising quantico (Ising com campo transverso) com uma impureza foi solucionado de forma exata. Este sistema de spins pode ser simulado de forma analítica por partículas quânticas (transformação de Jordan-Wigner). Caracterizamos o espectro, as autofunções e a evolução temporal da magnetização para estados iniciais particulares, focando no papel desempenhado pelos estados de impureza. Finalmente observamos oscilações remanescentes na magnetização, após a relaxação do sistema, para alguns valores dos parâmetros da impureza nos quais existem dois estados ligados no espectro de energias
Abstract: The description of dynamics of quantum many-body systems is a key ingredient to perform quantum computation and/or simulations of quantum behavior. In the present proposal, we study the time evolution of quantum spin chains with impurities at one of the boundaries, in order to understand the role of defects in relaxation properties. The quantum (transverse) Ising model with an impurity has been solved in exact form, using the Jordan-Wigner transformation, where spins are mapped onto spinless fermions, thus simulating analytically a spin system with particles. We completely characterize the spectrum, with the presence of bound states depending on values of the impurity parameters. We calculate the local magnetization and observe its relaxation for particular non-homogeneous initial states. Surprisingly, remanent Rabi oscillations are observed at asymptotically long times, when the spectrum displays two bound states
Mestrado
Física
Mestre em Física
1247646/2013
CAPES
37
Hernández, José Javier Cerda. "Ising and Potts model coupled to Lorentzian triangulations." Universidade de São Paulo, 2014. http://www.teses.usp.br/teses/disponiveis/45/45133/tde-18032015-170430/.
Full textAbstract:
The main objective of the present thesis is to investigate: What are the properties of the Ising and Potts model coupled to a CDT emsemble? For that objective, we used two methods: (1) transfer matrix formalism and Krein-Rutman theory. (2) FK representation of the q -state Potts model on CDTs and dual CDTs. Transfer matrix formalism permite us to obtain spectral properties of the transfer matrix using the Krein-Rutman theorem [KR48] on operators preserving the cone of positive func- tions. This yields results on convergence and asymptotic properties of the partition function and the Gibbs measure and allows us to determine regions in the parameter quarter-plane where the free energy converges. Second methods permite us to determine a region in the quadrant of parameters , > 0 where the critical curve for the classical model can be located. We also provide lower and upper bounds for the innite-volume free energy. Finally, using arguments of duality on graph theory and hight-T expansion we study the Potts model coupled to CDTs. This approach permite us to improve the results obtained for Ising model and obtain lower and upper bounds for the critical curve and free energy. Moreover, we obtain an approximation of the maximal eigenvalue of the transfer matrix at lower temperature.O objetivo principal da presente tese é pesquisar : Quais são as propriedades do modelo de Ising e Potts acoplado ao emsemble de CDT? Para estudar o modelo usamos dois métodos: (1) Matriz de transferência e Teorema de Krein-Rutman. (2) Representação FK para o modelo de Potts sobre CDT e dual de CDT. Matriz de transferência permite obter propriedades espectrais da Matriz de transferência utilizando o Teorema de Krein-Rutman [KR48] sobre operadores que conservam o cone de funções positivas. Também obtemos propriedades asintóticas da função de partição e das medidas de Gibbs. Esses propriedades permitem obter uma região onde a energia livre converge. O segundo método permite obter uma região onde a curva crítica do modelo pode estar localizada. Além disso, também obtemos uma cota superior e inferior para a energia livre a volume infinito. Finalmente, utilizando argumentos de dualidade em grafos e expansão em alta temperatura estudamos o modelo de Potts acoplado as triangulações causais. Essa abordagem permite generalizar o modelo, melhorar os resultados obtidos para o modelo de Ising e obter novas cotas, superior e inferior, para a energia livre e para a curva crítica. Além disso, obtemos uma aproximação do autovalor maximal do operador de transferência a baixa temperatura.
38
Navarrete, Manuel Alejandro Gonzalez. "Modelo de Ising ferromagnético com campo externo periódico." Universidade de São Paulo, 2015. http://www.teses.usp.br/teses/disponiveis/45/45133/tde-28082015-000711/.
Full textAbstract:
Estudamos o diagrama de fases para uma classe de modelos de Ising ferromagnéticos em $ \\mathbb^2 $, com campo magnético externo periódico. O campo externo assume dois valores: $ h $ e $ -h $, onde $ h> 0 $. Os sítios associados a valores positivos e negativos do campo externo, formam uma configuração em forma de tabuleiro de xadrez (nós chamamos de {\\it cell-board configuration}), com células retangulares de tamanho $ L_1 \\times L_2 $ sítios, de tal forma que o valor total do campo externo é zero. Como principal resultado, mostramos a presença de uma transição de fase de primeira ordem. A transição de fase existe para $ h <\\frac + \\frac $, onde $ J $ é uma constante de interação. A prova é construida usando o método de {\\it reflection positivity (RP)}. Aplicamos uma desigualdade que é normalmente referida como a estimativa de {\\it chessboard}. Além disso, incluímos uma região de unicidade da medida de Gibbs em $h>4J$, isto usando um critério baseado nas ideias de percolação em desacordo.We study the low-temperature phase diagram for a ferromagnetic Ising model on $\\mathbb^2$, with a periodical external magnetic field. The external field takes two values: $h$ and $-h$, where $h>0$. The sites associated with positive and negative values of external field form a cell-board configuration with rectangular cells of sides $L_1\\times L_2$ sites, such that the total value of the external field is zero. As a main result, we show the presence of a first-order phase transition. The phase transition holds if $h<\\frac+ \\frac$, where $J$ is an interaction constant. We use the reflection positivity (RP) method. We apply a key inequality which is usually referred to as the chessboard estimate. Furthermore, we prove uniqueness for Gibbs measure in $h>4J$, using a uniqueness condition obtained in terms of disagreement percolation.
39
Santos, Ricardo Paupitz Barbosa dos. "Modelo de Ising diluído na rede de Bethe." Universidade de São Paulo, 2002. http://www.teses.usp.br/teses/disponiveis/43/43134/tde-09082017-155024/.
Full textAbstract:
Estudamos o modelo de Ising com diluição de sítios numa rede de Bethe. a estrutura hierárquica da rede de Bethe leva de forma natural às relações de recorrência satisfeitas pelas distribuições de probabilidade dos campos efetivos. As quantidades termodinâmicas na rede de Bethe são então expressas explicitamente em termos das distribuições limite dos campos efetivos. As distribuições dos campos efetivos em T=0 são obtidas de forma numericamente exata (isto é, se desprezarmos os erros de arrendodamento) e também analiticamente em alguns casos selecionados. Encontramos no caso de interações ferromagnéticas um número sempre finito de campos efetivos possíveis, mas no caso de interações antiferromagnéticas esse número pode divergir para valores irracionais do campo aplicado. Esses resultados fornecem o diagrama de fases campo aplicado versus concentração, numericamente exato, para antiferromagnetismo diluído em T=0. As distribuições dos campos efetivos são determinadas aproximadamente para T>0 e utilizadas para o cálculo de diferentes grandezas termodinâmicas. Apresentamos as curvas de magnetização, energia livre, energia interna e entropia. Esses cálculos fornecem o diagrama de fases aproximado no espaço tridimensional de campo aplicado, temperatura e concentração.The site diluted Ising model is studied on a Beth lattice. The hierarchical structure of the Bethe lattice leads naturally to recursion relations obeyed by the probability distributions of the effective fields. The thermodynamic quantities on the Bethe lattice are then explicitly written in terms of the limiting distributions of the effective fields. Numerically exact results (i.e. if we neglect roundoff errors) for the distributions of the effective fields for T = 0 are presented, together with analytic results for select cases. It is found that the number of effective fields is always finite in the case of ferromagnetic interactions , but it might diverge for irrational values of the applied field in the case of antiferromagnetic interactions. These results yeld a numerically exact applied field versus concentration phase diagram for diluted antiferromagnet at T = 0. The distributions of the effective fields are computed aproximately for T > 0 and used to evaluete various thermodynamic quantities. Curves for the magnetization, free energy, internal energy and entropy are displayed. These calculations give an approximate three-dimensional phase diagram in the space of applied field, temperature and concentration.
40
Júnior, José Emílio de Lucena. "Modelo de Ising aplicado ao estudo da criminalidade." Universidade de São Paulo, 2014. http://www.teses.usp.br/teses/disponiveis/100/100132/tde-02042015-160340/.
Full textAbstract:
Nosso estudo foi elaborado a partir de uma analogia do modelo de Ising em duas dimensões para analisar a influência que a rede de contatos e as forças externas podem exercer no indivíduo para que ele tenha ou não a intenção de agir licitamente. Esse estudo teve como inspiração o modelo proposto no artigo Analysing Tax Evasion Dynamics Via The Ising Model (ZAKLAN; WESTERHOFF; STAUFFER, 2009), porém, com ênfase à intenção dos agentes, que precede a conduta delituosa, e não ao cometimento do crime em si, quando já ocorreu o dano à sociedade. A analogia e inclusão de algumas variáveis ao referido modelo nos possibilitou estudar, de acordo com cada cenário, formas de manter ou reduzir os índices criminais, prever possíveis situações de histerese, suas consequências e possíveis custos para a sociedade e para o governo.Our study was drawn from an analogy of the Ising model in two dimensions to analyze the influence that the network and the external forces can exert on the individual so that whether or not he intends to act lawfully. This study was inspired by that proposed in the article \"Analysing Tax Evasion Dynamics Via The Ising Model\" (ZAKLAN; WESTERHOFF; STAUFFER, 2009) model, but with emphasis on the intention of the agents, which precedes the criminal conduct, not to the commission of the crime itself, when the damage has already occurred to society. The analogy is the inclusion of some variables that model allowed us to study, according to each scenario, ways to maintain or reduce crime rates, predict possible situations hysteresis, their consequences and potential costs to society and the government.
41
Frazão, Camila Ribeiro. "Modelo de Ising em sistemas núcleo/casca nanomagnéticos." reponame:Repositório Institucional da UnB, 2014. http://repositorio.unb.br/handle/10482/17063.
Full textAbstract:
Dissertação (mestrado)–Universidade de Brasília, Faculdade UnB de Planaltina, Mestrado em Ciências de Materiais, 2014.Submitted by Ana Cristina Barbosa da Silva (annabds@hotmail.com) on 2014-11-25T15:19:10Z No. of bitstreams: 1 2014_CamilaRibeiroFrazao.pdf: 2148462 bytes, checksum: 736693a12406451fd34fa93a41a72f4e (MD5)
Approved for entry into archive by Patrícia Nunes da Silva(patricia@bce.unb.br) on 2014-11-26T11:42:46Z (GMT) No. of bitstreams: 1 2014_CamilaRibeiroFrazao.pdf: 2148462 bytes, checksum: 736693a12406451fd34fa93a41a72f4e (MD5)
Made available in DSpace on 2014-11-26T11:42:46Z (GMT). No. of bitstreams: 1 2014_CamilaRibeiroFrazao.pdf: 2148462 bytes, checksum: 736693a12406451fd34fa93a41a72f4e (MD5)
Neste trabalho foi realizada a investigação do diagrama de fase de partículas presentes em sistemas magnéticos nanoscópicos influenciados por capas que respondem à exposição de campos magnéticos. Foi utilizado o Modelo de Ising para representar o sistema magnético e o Método Monte Carlo com o Algoritmo de Metrópolis para obtenção das grandezas físicas mais relevantes. Levou-se em conta 4 diferentes estruturas, 3 bidimensionais: quadrada, triangular, tipo colmeia e 1 tridimensional: cúbica. Considerou-se apenas a influência dos primeiros vizinhos na rede e foi utilizado um modelo diferenciado de distribuição de intensidades dos momentos magnéticos ao longo da rede. A análise das curvas das propriedades magnéticas das estruturas, em particular das medidas da suscetibilidade magnética e do calor específico, permitiram identificar sucessivas transições de fase em baixas temperaturas. As transições de fase referentes às camadas da rede na simulação possibilitaram a elucidação do comportamento das curvas de propriedades magnéticas encontradas experimentalmente. _________________________________________________________________________________ ABSTRACT
In this work was made an investigation about the particle phase diagramresident in nanoscopic magnetic fields influenced by cases that respond toexposition of magnetic fields. The Ising model was used to represent the magneticsystem and the Monte Carlo method with the Metropolis algorithm to obtain themost relevant physical quantities. We took into account four different structures:square, triangular, honeycomb and cubic, taking into account just the influence ofthe first neighbors in the network and using a different model of intensitydistribution of the magnetic moments along the network. The analysis of thecurves of the magnetic properties of the structures, in particular of themeasurements of the magnetic susceptibility and of the specific heat, allowed usto identify successive phase transitions in low temperatures. The phase transitionsrelated to the network layers in the simulation allowed the elucidation about thebehavior of the curves of the magnetic properties founded throughexperimentation.
42
Ye, Feng. "Random-field ising ordering above magnetic vacancy percolation /." Diss., Digital Dissertations Database. Restricted to UC campuses, 2003. http://uclibs.org/PID/11984.
Full text
43
Moreira, Antonio Flavio Barbosa. "Magnetismo de superficie em sistemas compressiveis de Ising." reponame:Repositório Institucional da UFSC, 1991. http://repositorio.ufsc.br/xmlui/handle/123456789/75718.
Full textAbstract:
Dissertação (mestrado) - Universidade Federal de Santa Catarina. Centro de Ciencias Fisicas e MatematicasMade available in DSpace on 2012-10-16T03:47:45Z (GMT). No. of bitstreams: 0Bitstream added on 2016-01-08T17:10:48Z : No. of bitstreams: 1 84767.pdf: 1765574 bytes, checksum: 20023fc08eb7998d91be1d5d091296e5 (MD5)
Neste trabaho estudamos o modelo de Ising em uma rede cúbica semi-infinita. Consideramos um modelo magneto-elástico com uma pressão uniaxial, onde os íons vibram somente em uma direção perpendicular aos planos cristalinos. Na aproximação de campo médio determinamos o diagrama de fases para os acoplamentos críticos de superfície em função da pressão, e o perfil da magnetização. Utilizando o grupo de renormalização de campo médio determinamos as superfícies críticas do modelo de Ising na rede cúbica semi-infinita considerando blocos de até 25 spins. O resultados que obtivemos para o acoplamento crítico de superfície é comparável àquele obtido através de simulação de Monte Carlo nesse mesmo modelo.
44
Santos, Marcio. "Transições de fase em modelos de ising cineticos." reponame:Repositório Institucional da UFSC, 1994. http://repositorio.ufsc.br/xmlui/handle/123456789/76022.
Full textAbstract:
Dissertação (mestrado) - Universidade Federal de Santa Catarina, Centro de Ciencias Fisicas e MatematicasMade available in DSpace on 2012-10-16T06:39:06Z (GMT). No. of bitstreams: 0Bitstream added on 2016-01-08T19:13:28Z : No. of bitstreams: 1 97845.pdf: 1679736 bytes, checksum: 7ce84e5cb3ceda57783814ee7b99fcc2 (MD5)
Neste trabalho estudamos dois problemas relativos à transição de fases em modelos magnéticos através da equação Mestra. No primeiro deles, consideramos a evolução, em direção ao estado estacionário, de uma cadeia dupla de spins, através da relaxação inicial do parâmetro de ordem. Mostramos que o expoente crítico dinâmico pode depender dos aspectos microscópicos da Hamiltoniana, não exibindo um caráter universal, para as taxas de transição de Glauber e Kawasaki. No segundo problema determinamos os estados estacionários para o modelo de Ising anisotrópico em duas dimensões, levando-se em conta a correlação entre primeiros vizinhos. Determinamos o diagrama de fases desse modelo, que apresenta as fases antiferro, ferro e paramagnética.
45
Campos, Paulo Roberto de Araujo. "Comportamento crítico dinâmico de algoritmo de Wolff no Modelo de Ising com correlação de sítios e ligações." Universidade de São Paulo, 1998. http://www.teses.usp.br/teses/disponiveis/76/76131/tde-10092008-092104/.
Full textAbstract:
Estudamos o comportamento dinâmico do algoritmo de Wolff no modelo de Ising diluído com correlação de sítios e ligações (modelo SBC). Nosso objetivo principal foi estudar a performance deste algoritmo em um sistema onde além da desordem à presença de impurezas não magnéticas, houvesse mais um parâmetro presente, a correlação espacial. Além disto foi possível obter o diagrama de fases para o modelo, o qual possibilita entendermos um pouco o efeito da desordem e da correlação no sistema.Verificamos que o diagrama de fases por nós obtidos está em boa concordância com os dados experimentais obtidos com o composto magnético Knip Mg1-pF3, o qual foi a motivação para o modelo SBC. O estudo do comportamento dinâmico nos possibilitou entender um pouco mais como o algoritmo de Wolff se comporta quando submetido a sistemas mais complexos, como é o caso do modelo em estudo, verificamos uma melhor performance deste algoritmo à medida que tanto a diluição quanto a correlação é aumentada. Esse comportamento é oposto àquele verificado nos algoritmos locais. Essa melhor performance do algoritmo de Wolff quando submetido a tais sistemas é bastante positivo, pois isto possibilita obtermos medidas de quantidades físicas de interesse de forma mais precisa, pois há uma redução drástica da correlação estatística entre configurações produzidas por esta dinâmica.We extend the Wolff Algorithm to include correlated spin interactions in diluted magnetic systems. This algorithm is applied to study the site-bond-correlated Ising model on a two-dimensional square lattice. We use a finite-size scaling procedure to obtain the phase diagram in the temperature-concentration space. Our results are in excellent agreement with the experimental data for the Knip Mg1-pF3, compound. We also present the critical dynamical behavior of the Wolff algorithm for this system. We have verified that the autocorrelation time diminishes in the presence of dilution and correlation, showing that the Wolff algorithm performs even better in such situations. This behavior is completely different from those exhibited by the single spin-flips algorithms.
46
Dillmann, Oliver. "Monte-Carlo-Simulationen zum kritischen Verhalten dünner Ising-Filme." [S.l.] : [s.n.], 2000. http://ArchiMeD.uni-mainz.de/pub/2000/0071/diss.pdf.
Full text
47
Gibson, Sarah. "Merchant-ising England : the cultural consumption of the Englishness." Thesis, Lancaster University, 2003. http://ethos.bl.uk/OrderDetails.do?uin=uk.bl.ethos.423929.
Full text
48
Eckner, Sinziana Maria. "Stochastic Ising Models at Zero Temperature on Various Graphs." Thesis, New York University, 2014. http://pqdtopen.proquest.com/#viewpdf?dispub=3665138.
Full textAbstract:
In this thesis we study continuous time Markov processes whose state space consists of an assignment of +1 or -1 to each vertex x of a graph G. We will consider two processes, σ( t) and σ'(t), having similar update rules. The process σ(t) starts from an initial spin configuration chosen from a Bernoulli product measure with density θ of +1 spins, and updates the spin at each vertex, σx(t), by taking the value of a majority of x's nearest neighbors or else tossing a fair coin in case of a tie. The process σ'( t) starts from an arbitrary initial configuration and evolves according to the same rules as σ(t), except for some vertices which are frozen plus (resp., minus) with density ρ+ (resp., & ρ–) and whose value is not allowed to change. Our results are for when σ(t) evolves on graphs related to homogeneous trees of degree K ≥ 3, such as finite or infinite stacks of such trees, while the process σ'(t) evolves on Zd, d ≥ 2. We study the long time behavior of these processes and, in the case of σ'(t), the prevalence of vertices that are (eventually) fixed plus or fixed minus or flippers (changing forever). We prove that, if θ is close enough to 1, σ(t) reaches fixation to +1 consensus. For σ'( t) we prove that, if ρ+>0 and ρ– = 0, all vertices end up as fixed plus, while for ρ+ >0 and ρ– very small (compared to ρ +), the fixed minus and flippers together do not percolate.
49
Jiménez, Ramírez Andrea Patricia. "Estados Satisfactorios del Modelo de Ising Antiferromagnético en Triangulaciones." Tesis, Universidad de Chile, 2012. http://www.repositorio.uchile.cl/handle/2250/102786.
Full text
50
Filho, Raimundo Valmir Leite. "Impurezas MagnÃticas em Ferromagnetos de Ising com Campo Transverso." Universidade Federal do CearÃ, 2005. http://www.teses.ufc.br/tde_busca/arquivo.php?codArquivo=2917.
Full textAbstract:
Conselho Nacional de Desenvolvimento CientÃfico e TecnolÃgicoFundaÃÃo de Amparo à Pesquisa do Estado do CearÃ
O formalismo de funÃÃes de Green à usado para calcular o espectro de excitaÃÃes associados a impurezas magnÃticas implantadas em um filme ferromagnÃtico descrito pelo modelo de Ising com campo transverso. AtravÃs do mÃtodo da equaÃÃo de movimento expressÃes explÃcitas para as funÃÃes de Green sÃo determinadas para um ferromagneto sem impurezas. A funÃÃo de Green para um filme ferromagnÃtico contendo impurezas à obtida atravÃs da equaÃÃo de Dyson. O espectro de ondas de spin relativo Ãs impurezas à obtido para freqÃÃncias abaixo do limite inferior da banda de volume para um material puro. Com o objetivo de avaliar a influÃncia da posiÃÃo das impurezas no filme no espectro de excitaÃÃes, consideramos trÃs diferentes disposiÃÃes geomÃtricas para as impurezas: linha de impurezas perpendicular à superfÃcie do filme, quatro impurezas em um plano paralelo `as superfÃcies e quatro impurezas em um plano perpendicular `as superfÃcies do filme. Obtemos resultados para a freqÃÃncia dos modos localizados como funÃÃo do parÃmetro de troca entre duas impurezas vizinhas, do parÃmetro de troca entre as impurezas e seus vizinhos e do parÃmetro de campo efetivo nas impurezas.
A Green function formalism is used to calculate the spectrum of excitations associated with magnetic impurities implanted in a ferromagnetic thin film described by the transverse Ising model. Using the equations of motion method, explicit expressions for the Green function are determined for a ferromagnetic without impurities. The Greenâs functions for a ferromagnetic film containing impurities are obtained through Dyson equation. We consider only the âdefectâ modes that appear below the bulk band of the pure material. In order to assess the influence of the position of the impurities in the film on the excitations spectra, we consider three different geometrical arrangement for the impurities: impurities line perpendicular to the surface film, four impurities in a plane paralel to the surface, and four impurities in a plane perpendicular to the surfaces of the film. We obtain results for the frequencies localized modes as a function of the exchange parameter between two impurities neighborings, of the exchange parameter between the impurities and their neighborings, and the effective field parameter at the impurities.